LOFT: Localized, Fusion and Tensors of Frames
Representing operators is an important part of operator theory, also essential for the numerical solution of operator equations. Traditionally, orthonormal bases have been used to obtain matrix representations while recently frames have entered the picture. The quality of the matrix representation depends crucially on the chosen class of frames.
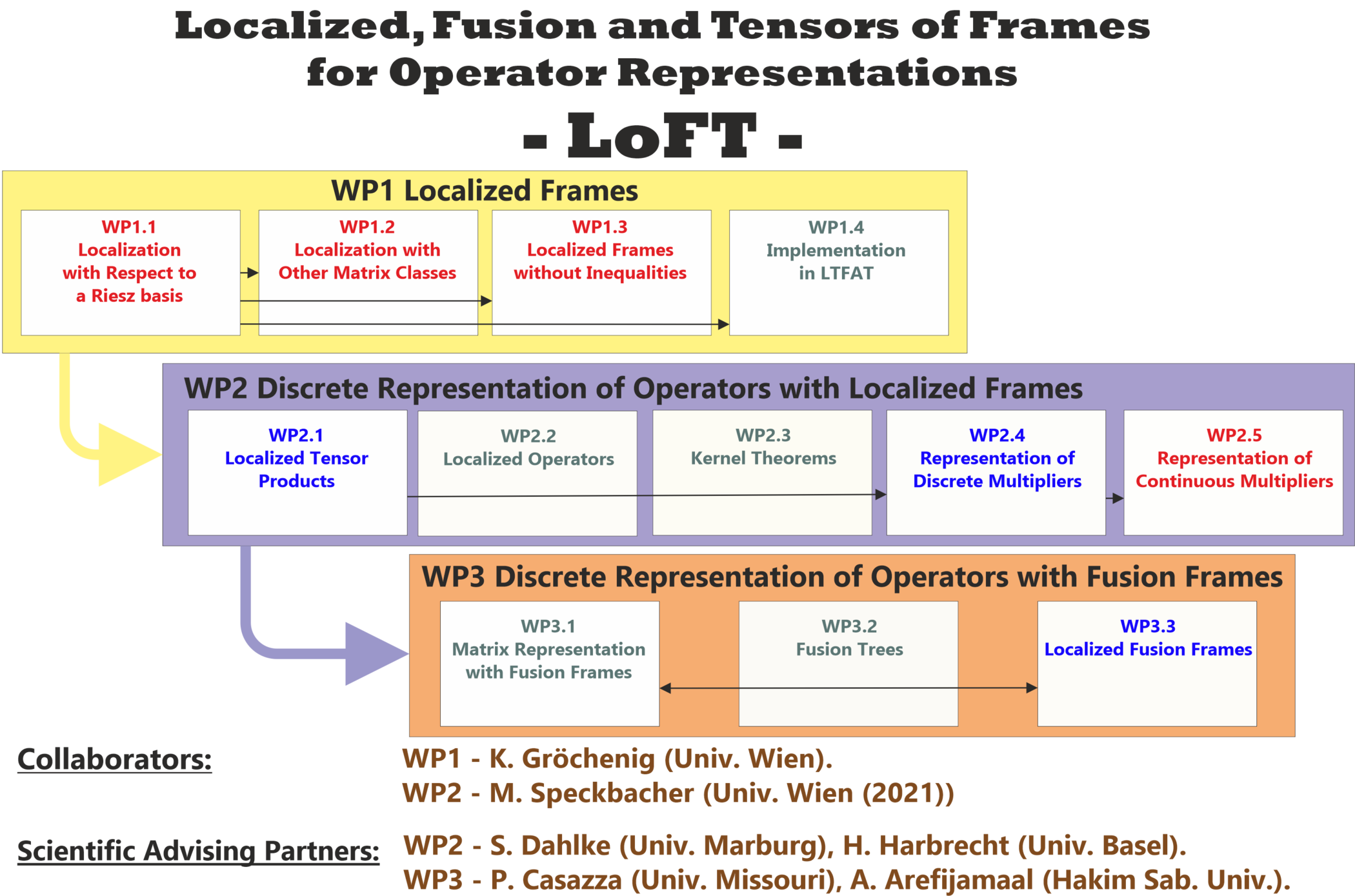
One particularly promising approach, to describe 'good' frames, is the concept of localized frames. The investigation of representations of operators with those frames has recently been started, supporting the important role of tensor products of frames in those representations. Finally, fusion frames are naturally linked to domain decomposition methods for the integral representation of operators.
Operator representations with those three classes is still a largely unexplored field in frame theory.
We will advance the mathematical theory of localized frames, fusion frames and their tensor products, for the representation of operators, connecting harmonic analysis and operator theory.
For localized frames we will investigate the link between frames that are localized with respect to a fixed Riesz basis and intrinsically localized frames, show frame properties without inequalities, use more general localization matrix algebras, and implement them in the open source toolbox LTFAT.
For tensors of frames we investigate when they form localized systems, when operators are localized, related kernel theorems, and the representation of discrete and continuous multipliers.
Finally, for fusion frames we look at the related matrix representations, and how they can be combined with a tree structure, as well as the localization concept.
This project was granted as a 3-years FWF project, that will start approximately in October 2021, hiring three PhDs.
Externe Partner
- Arefijamaal, Ali Akbar (Hakim Sabzevari University)
- Casazza, Peter (University of Missouri)
- Dahlke, Stephan (Philipps-Universität Marburg)
- Gröchenig, Karlheinz (Universität Wien)
- Harbrecht, Helmut (Universität Basel)
Finanzierung
FWF - P 34624

